We had a very interesting and engaging session
with the very energetic Peggy this morning. There were many hands on activities
that we had. My favourite activity was the paper art activity. It was an
activity that encourages us to think and predict. We used the 3-column method
to present out ideas on the outcome of four fold triangle. We wrote down what
we see, think and wonder.
One of my biggest takeaways from this
lesson was the ‘using stories to teach mathematics’. I learn new activities
that could be included in the before reading activity. For example, we could
make word cards of the different characters from the story book and get the
children to predict the story. The children can create a story by taking turns
to come out with a sentence using the character word card. After telling the
story, they can also tell the retell the story using the word cards.
From the story, we design a mathematics task. This was when we talked about differentiation. Many times, we always
forget the importance of differentiating our lessons for young children. There
are three ways in which we can differentiate lessons – by content, by process
and by product.
The session ended with Peggy talking about lesson
study – professional development process/tool that teachers engage in to
systematically examine their processes. I think that this is very important to
have in every school. For this to happen successfully, it needs to be
teacher-driven/initiated, job-embedded and collaborative learning.
I truly enjoyed all my 6 sessions of
elementary mathematics. And like I mentioned in my first session, my entire
perspective of mathematics has changed. The sessions reminded me of the
implications of the approaches I adopt when teaching mathematics to young
children. Out with the old and in with the new!
.JPG)

.JPG)

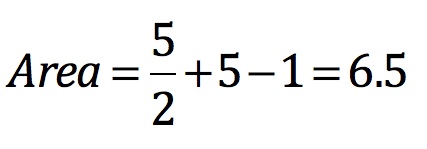

.JPG)
.JPG)

